Malenaadu: Principles of cellular frequency reuse

In the cellular concept, frequencies allocated to the service are re-used in a regular pattern of areas, called 'cells', each covered by one base station. In mobile-telephone nets these cells are usually hexagonal. In radio broadcasting, a similar concept has been developed based on rhombic cells.
To ensure that the mutual interference between users remains below a harmful level, adjacent cells use different frequencies. In fact, a set of C different frequencies {f₁, ..., f_C} are used for each cluster of C adjacent cells. Cluster patterns and the corresponding frequencies are re-used in a regular pattern over the entire service area.

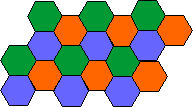Frequency reuse plan for C = 3, with hexagonal cells. (i=1, j =1)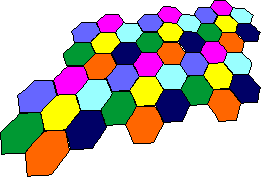

Frequency reuse plan for C = 7 (i=2, j =1).

The total bandwidth for the system is C times the bandwidth occupied by a single cell.

Real-World Cells

In the practice of cell planning, cells are not hexagonal as in the theoretical studies. Computer methods are being used for optimised planning of base station location and cell frequencies. Pathloss and link budgets are computed from the terrain features and antenna data. This determines to coverage of each base station and interference to other cells.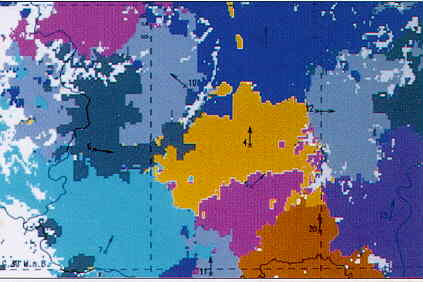

Source: Siemens TORNADO D Cellular Planning Tool

Reuse Distance

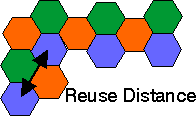The closest distance between the centres of two cells using the same frequency (in different clusters) is determined by the choice of the cluster size C and the lay-out of the cell cluster. This distance is called the frequency 're-use' distance. It can be shown that the reuse distance r_u, normalised to the size of each hexagon, is

r_u = SQRT{3 C}

For hexagonal cells, i.e., with 'honeycomb' cell lay-outs commonly used in mobile radio, possible cluster sizes are C = i² + ij + j², with integer i and j(C = 1, 3, 4, 7, 9, ...). Integers i and j determine the relative location of co-channel cells.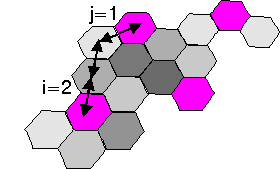

7-cell reuse with i = 2 and j =1.

Derivation

Listen to an animated audio presentation by Jean-Paul Linnartz (embedded html, SMIL, PPT-only)
Also, for more details on the math behind hexagonal cell layouts, check this pdf document.

Exercise

Show that r_u = SQRT {3(i² + ij + j²)}.
Compute the surface area of a cluster of cells, both in terms of

Cluster size C and the size of a single cell, and
i and j and the size of a single cell.

Use these two expressions to verify that C = i² + ij + j². Answer.

In practical FM or digital cellular networks for public radio telephony, the cluster size mostly is on the order of C = 7 or 9, though with special techniques, such as diversity reception, smaller re-use distances can be used. GSM can work with C = 3 or 4.
Cellular CDMA systems can use C = 1, i.e., the same frequency is used in all cells.

Spectrum Efficiency

In most cellular systems, each base station can carry more than one telephone call in its cell. If the number of parallel channels per base station is denoted by M, the total bandwidth for the cellular net B_s is the product of the occupied bandwidth per channel B_T, the number of channels per cell M and the cluster size C. Thus B_s = M C B_T. The spectrum efficiency S_E of a cellular net can be defined as the carried traffic per cell A_c, expressed in erlang, divided by the bandwidth of the total system B_s and divided by the area of a cell S_u. So


           A_c     
SE =  ----------- 
      B_T C M S_u

Here, A_c is mostly computed from Erlang B formulas, with A_c equals the attempted traffic multiplied by the probability of success (= 1 - blocking probability).Mostly, the spectrum efficiency is expressed in erlang/MHz/km2.
Thus, we observe that the spectrum efficiency decreases with the cluster size C. System performance, for instance expressed in terms of the outage probability or the bit error rate experienced by the user, improves with increasing re-use distance, so it improves with the cluster size. Hence, achieving high system performance and efficient use of the radio spectrum are conflicting objectives for a network designer.